Light is not a Sine Wave but a Particle with Spin
(from MM: The Wavelength and Frequency of Light are Reversed)
© Miles Mathis Abstract: Wavelength and frequency of light have been misassigned for centuries. Having abandoned the existence of ether, it is ludicrous to assign light as a field wave. Light is still being diagramed as a sine wave like sound, which is a field wave, rather than explaining how the actual spin motion of the photon mimics a wave. In this section of his paper, Miles Mathis will show how the equation c = λυ is incomplete and then will rewrite the photon energy equations by
dissolving Planck's constant h out of them altogether. (The Parts 1 & 2: History and Diffraction Grating sections are not shown here.) Light as a real particle, with real radius and real mass. It also has real spin. In fact, it has
several spins. These spins stack to create the characteristics of light we experience. What this means is
that light is spinning photons . Light is not a particle/wave. It is a particle with a spin. This spin can
then create waves in data or fields, but the waves do not belong to the photon. The current waves are
field results, as in the interferometer. There is no wave/particle duality. The photon is a particle that
may (or may not) create a wave in the data. In the photoelectric effect, there is no wave in the data,
which is precisely why we say the light is a particle in that experiment. In looking at the the following animations, it can be seen how
the true spin wave of a photon can be turned into a sine wave diagram. Download Chris
Wheeler's animation:
What you will see is that if we create a sine wave from spin motion, it is actually the frequency of the
spin that creates the wavelength of the sine wave , thus the frequency determines the length of the
wave.
Using a point on the circumference of the spin, it can be seen that the gap between wavecrests is the
time it takes that point to make one rotation. So the length of the sine wave is caused by the frequency
of the spin! When we think we are measuring wavelength, we are really measuring frequency.
Note that this is not to say that the sine wavelength is function of the frequency as
current theory says, but that the sine
wavelength is created by the spin velocity. More than that:
although current diagrams get a sine wavelength from a spin velocity—there is in fact no sine
wavelength. The sine wave does not exist in real life because there is no field . We cannot assign our
“wavelength” to a sine wave on a diagram, because you cannot diagram something that does not exist.
The only thing that exists is the spin of the photon, which is a frequency, not a wavelength. The
wavelength is not a function of the frequency; the wavelength is manufactured in the diagram from the
frequency. The frequency exists, the wavelength does not.
In other words, we are currently assigning the variable λ in the equation c = λυ to something that does not exist. We are
assigning it to the wavelength on a sine diagram, but that diagram is not applicable to the real field.
The photon doesn't have a wavelength of that sort .
And what we currently call the frequency of light is also manufactured, since it comes from the sine-
wave diagram as well. Currently, the frequency of light is actually the frequency of the sine wave on
the sheet of paper, not the frequency of the photon spin. They aren't the same thing, as we will see
below.
Our current variables are assigned to a manufactured sine wave, but physically, light
is a spin wave, not a sine wave. The photon itself is moving as a spin wave, not as a pattern on a
background. (See The Error of the M/M Interferometer.) Current theory hides out in frequency with light because it has no way of calculating spin velocities. (See Unifying the Photon with other Quanta, How they Travel and why they go c.)
In fact current theory does not even understand the difference between tangential velocity and orbital velocity. (See A Correction to Newton's Equation a=v2/r.)
This is why the mainstream prefers to talk of frequencies, but frequencies are
imprecise things. Spins with completely different radii can accidentally have equal frequencies. To
really know what is going on, we need both a frequency and a radius, which will give us a velocity.
Since MM has now calculated a radius for our photon, we do not need to hide away in frequencies. We
can talk about velocities instead.
We need to get into spin velocity here instead of frequency, because although you can calculate one
from the other very easily, they aren't necessarily proportional. If the radius was constant, then, if
you increase a spin velocity, you will increase the frequency. However, we are not keeping the radius constant
because as the energy increases, so does
the radius of our photon. If we increase the radius and keep the same frequency, the spin
velocity must rise. We could even increase the radius, lower the frequency, and the spin velocity would
still rise. So the basic problem is that the current equations do not include any of that complexity,
because they do not take into account the changing spin radius.
The equation c = λυ is too simple because it includes neither the spin velocity of the photon nor its radius. It is
completely opaque to any and all mechanics. It applies to the old field waves, like sound. It
does not apply to light, because it has no way of representing the increasing radius of the photon as we
move up from infrared to ultraviolet. That equation applies only to a point source, or at best to a
particle with a constant radius. It cannot be applied to our photon, since it has no way to include the
changing radius. It is for this reason that the mainstream has resist giving the photon size and why they want it to be a point.
What we have to do is replace frequency with velocity, jettison the equation c = λυ We do not have a way to measure the frequency or wavelength of
light directly. Frequency , which is simply a derived number, taken from the
the equation c = λυ. Wavelength is also a derived
number. It it is measured “directly from data” from machines like
interferometers, but it is not the wavelength being measured in the data. It is “measured” by counting
the number of fringes or something like that. The physicists then simply assume that the fringes are
caused by wavelength, and they find a way to develop a number for that wavelength, but since this sine wavelength on their diagrams does not exist. What is causing the fringes is not
sine wavelengths, it is the velocity of spins with given radii. The only “wavelength” involved is the
spin radius, and that is not what they mean by wavelength. The radius of the photon never enters current
equations. because they still believe the photon is a point particle.
To say this in another way, even interferometry and laser mixing require assumptions and math to get a
wavelength. We cannot just lay a ruler on the data and get the wavelength of the light. The data is not a
direct measurement of the photon. It is a result of the photon interacting with the machine or other
photons. All our measurements of the photon are indirect measurements, and since indirect
measurements require math and field assumptions, they are prone to error. That is what is happening
here. The equations and assumptions are wrong, so the numbers out are all wrong.
To be specific, it has always been assumed that shorter wavelength light will be diverted or bent more
by prisms and other diffractors and refractors (including interferometers) because it has a short
wavelength. It turns out this is false as shown on MM's paper prism analysis an his site. Light is not bent at all. The
spectrum is created by the charge field “pushing” red light more than blue, and this is true in diffraction
just as in refraction. Because red is smaller, it is more easily pushed by the charge field. Violet is
actually diverted or bent the least , but the diagrams are misread. This is where the reversal takes place.
This is where the current theory is upside down. This false assumption had caused all physicists since
Newton to assign wavelengths upside down. The charts are backwards.
Rather than analyze the prism again, let us look at
the interferometer, since it has given us these current wavelengths: According to current theory, the interferometer splits a beam of light into two beams, delays one with
respect to the other, and then recombines them to get interference. Problem is, it is assumed that the
interference is an interference of wavelengths, when it is not. MM has just shown you that the interference
must be physically caused by spin interaction, not interference of wavelengths. Physically,
wavelengths cannot affect one another. How can one length affect another length? No, only motions can
interact or interfere, and the motion interacting here is photon spin.
The basic starting equation of the interferometer is λ = 2d/m, where m is the number of fringes and 2d
is the distance between 2 point sources of light observed. From that one equation it can be seen that
physicists are assuming that the fringes are being caused by a wavelength directly. To measure the
wavelength, they simply measure the fringes. This is naïve because the physicists have always just assumed that wavelengths are interfering even though
the wavelength of light has never been assigned to anything. It is assigned to a sine wave on a
diagram, but then the diagram is assigned to nothing . If that assumption is wrong, then this equation is
wrong.
What is actually happening in the interferometer is that the light is being split into two parts. One part
is forced to take a path slightly longer than the other, then the paths are recombined. Currently this is
said to cause a wavelength discrepancy, which shows up as interference or fringes. However, what is really
happening is that the spins are being thrown off, so that when the light is recombined, half of it is
incoherent regarding spin. If we treat the photons as Feynman did—as little clocks—half the clocks
will be pointing to 2 and half will be pointing to 3, say. (See MM's paper Feynman's
Shrink-and-turn Method on his site.) They will still have the same speed and energy,
but they will be out of spin phase. This solves this problem the same way it solved the partial
reflection problem , because it explains the mechanics beneath Feynman's sumovers. It matters where
in its spin cycle the photon is, because the photon is composed of stacked spins.
By knowing where in
its spin cycle the photon is, we can tell where the body of the photon is inside the spins. Consult Chris
Wheeler's animation again that was downloaded previously and you can see that the body of the photon is never at the center of the
spins. This explains why being at 2 in the spin cycle is not the same as being at 3. The stacked spins
give the photon a varying momentum. In other words, if the photon body is forward of center in
collision, the photon will act very slightly differently than if the photon body is behind center.
To visualize the interferometer problem, imagine all the photons recombined into one beam after being
reflected by the central mirror, traveling side by side once more as they approach the detector at the
end. As they move along, they constantly jostle one another. Since the photons do not all hit the same
spot on the mirror, they do not have precisely the same trajectory, and some variation is the result. So
they do not travel in perfectly parallel trajectories. In short, they jostle. Well, when they collide side-
to-side, the outer spins meet. This meeting of spins is what creates what we call interference. The
spins can damp or augment, creating what we call peaks and troughs. And the distance between peaks
and troughs in an interferometer will be determined by the relative difference in energy of the spins. If
we have photons with a fast spin rate (or a higher spin frequency), larger gaps in the data will be
produced. Larger fringe gaps will be the result.
Just think about it. Say we have two sources of light. Source A and source B. In the first, the photons
are spinning at a rate of 4. In the second, they are spinning at a rate of 2. Now we put the first light
through the interferometer. Say the interferometer creates a path difference of 25% (we are using fat
round numbers here, obviously). On one path, Aa, we will say the photons happen to arrive at the
detector just as they were emitted, so they are at 4. On the other path, Ab, the photons are 25% off their
spin rate, so they are at 3. The difference is 1. Now we put the light from source B through the same
interferometer, still set at 25%. On path Ba, the photons arrive unphased, and so they are at 2. The
phased photons Bb arrive at the detector at 1.5. The difference is .5. In other words, the interferometer
found a fringe of 1 with the first and .5 with the second. By current theory, they would assign the first
double the wavelength of the second, which is not correct.
In current theory, larger wavelength goes with lower frequency, but that is not what we
just found. Larger fringe gaps are therefore not caused by larger wavelengths. They are caused by
greater spin velocities. And greater spin velocities belong to smaller photons. And smaller photons
are less energetic.
This means current theory is both right and wrong. What they are calling long wavelength photons are
indeed less energetic photons. So they are right about the energies. But the less energetic photon does
not have either a long wavelength or a low frequency. It has a high spin frequency and a small radius.
It has nothing that we would call a wavelength, since no sine wave is created in the field. If we want to
assign a wavelength, we have to transform the radius into a wavelength.
To understand how a smaller, less energetic photon can have a higher
frequency has to do with radius. The redder photon has a smaller radius. This gives it a
smaller circumference. If the spin velocity is c, then the time of one rotation gives us a frequency.
That frequency must be greater than a photon with a larger radius. Hence, more spin velocity, less
energy. Just the opposite of what we are taught.
As an example of this, look at two planets in orbit. Give them the same local velocity v. If planet b has
a greater orbital radius than planet a, its period will be greater. Since frequency is 1/period, its
frequency will be less. It will return to the same spot less often.
All thus goes to show that we needed a radius in the wave equations, so
let us do the math. Since we have jettisoned the sine wavelength, we are free to re-assign the
variable λ to the radius. But rather than do that, let us assign it to how we would measure the radius
from our vantage. In other words, we will do a sort of Relativity transform on the radius, to find out
how we would measure it at our scale and speed. Since light is going c relative to us, c is the only
scaler we need. But since light has both a spin motion and a linear motion, we will need c twice in the
transform. If r is taken as a simple length, then that length is going to be stretched out by the motions
of the photon relative to us.
To see this, you have to go back to the Wiki animation I
borrowed, the one where the spin is being turned into a sine wave. That animation doesn't match our
photon, because we need to put our photon's spin in the same plane as its linear motion. We need to lay
the circle down and then move it along the x-axis, to even begin to get the right mechanics. So if we
have to try to diagram this, we diagram it this way instead:
Please note that this paper is a simplification by me of a paper or papers written and copyrighted by Miles Mathis on his site. I have replaced "I" and "my" with "MM" to show that he is talking. All links within the papers, not yet simplified, are linked directly to the Miles Mathis site and will appear in another tab. (It will be clear which of these are Miles Mathis originals because they will be still contain "I" and "my".) The original papers on his site are the ultimate and correct source. All contributions to his papers and ordering of his books should be made on his site.
(This paper incorporates sections of Miles Mathis' freq paper and some of freq3 paper.)
wave.wmv
Or a simpler animation, borrowed from Wikipedia, which shows the basic creation of a
sine wave from a spin, although it complicates the problem with sin and cos:
animated gif in a separate window.
(c = the speed of light
λ (Gr. letter lambda) is wavelength (meters)
υ (Gr. letter upsilon) is frequency in Hertz)
,
and reassign the
remaining variables to the photon instead of to the sine wave.
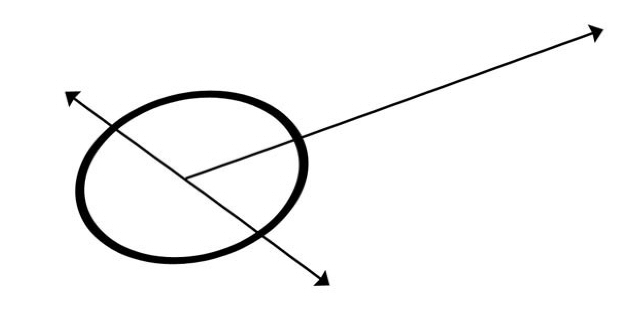
As you see, the circle doesn't travel standing up, as in the Wiki diagram. In my diagram, we put the spin radius directly in the line of motion, which must fully integrate r into all the energy equations.
It is clear that the radius will be stretched out by the linear motion of the photon, but it will also be stretched out by the spin motion. With the radius standing for a sort of baseline energy, the circumference will then have an energy relative to that radius, and the linear motion has an energy relative to r as well. So what we are doing here with this Relativity transform is transforming the local energy of the photon into the energy we measure. We are just letting the lengths stand for the energies, you see. And while the photon is gaining kinetic energy from its speed relative to us, c, it is also gaining kinetic energy from its spin. It is spinning while it is moving linearly, so we have to integrate the motions.
Using the finding that the circumference is 8 times the radius in kinematic situations as shown in MM's paper π (pi) is 4 not 3.14, then if we assume a point on the outer spin is going c, then we can find a total distance traveled in a given time and that the point also has a linear velocity c relative to us. So the total transform is just 8c2 . If the radius is taken as a local wavelength, then we will experience that wavelength as 8γrc2. (We are using c2 as a Relativity transform.)
Anyway, by simple substitution, the equation E = λυ becomes:
E = hc/8rγc2 But we can fine-tune and
simplify that as well.
We know that equation is in the wrong form, because the energy should be directly proportional to the radius. In that equation, it is inversely proportional. Since a larger particle at the same speed must have more energy, it is clear that the current equation is upside-down to that logic. If we insert a radius into the current equation, the radius is in the denominator! So, according to the current equation a larger photon would have a smaller energy. The current equation cannot be right. It is also clear that Planck's constant is skewing the equation. Since Planck's constant is just a fudge factor, we can also melt all the fudge out of the equation.
Putting the radius in the numerator where it has to be, then filling in the
rest to match current numbers, to stay mechanical, and following reason., hypothetically, let's try:
Eγ = 2rγ√c
If we use r = 2.74 x 10-24m from Unifying the Photon with other Quanta for the derivation of that radius), that equation differs from E = hc/8rγc2 by only 1.06, which is represented by this term: 4√4/π). We need that term because the current derivation for Planck's constant uses π when it should not. (See Planck's Constant and Quantization - a Mechanical Explanation and its Relation to the Mass of the Photon.) That is our transform to rectify the equation, taking π out of it. MM has used that equation before to rectify quantum equations in the paper The Stefan-Boltzmann Law proves E/M theory and the paper Newton's law is a Unified Field of Gravity and E/M and on the unified field ), so when MM saw the number 1.06, he knew immediately where it was coming from. It is the difference between 4 and π, at the fourth root. It is caused by treating quantum equations as static equations, when they are kinematic equations. In kinematic equations, we replace π with 4 according to the paper π (pi) is 4 not 3.14. This means that the standing photon energy equation with h in it is wrong by 6%.
In his paper Perihelion Precession of Mercury Explained MM showed that Einstein's field equations are 4% wrong in the field of the Sun, and now it turns out that the photon energy is off by 6%. (This is one reason the mainstream uses both Planck's constant and Dirac's constant. Because their equations are all messed up, they need both constants to help them fudge answers in a variety of problems.) They assure us these equations are correct to within a hair, but they never are. They are pushed into a ballpark by data, and the rest is just bluster.
|
We can now write photon mass and radius as functions of one another: Eγ = 2rγ√c = mγc2 mγ = 2rγ/c√c λ = 8E(rγ/mγ) = E(Cγ/mγ) C is circumference of the photon (z-spin) mγ = E(Cγ/λ) |
So MM has just shown where Planck's constant comes from, and dissolved it as well. We do not need it anymore. We can now get a photon energy straight from its radius or mass, or vice versa. Planck's constant was nothing more than mathematical residue from writing the energy in terms of the sine wave variables instead of the real variables of the photon like radius.
Conclusion
Correcting these longstanding errors will allow us a deeper understanding of the photon, and thereby all quantum interactions. In previous papers, MM has shown that this error has caused many problems, and the reversal of many explanations. For example, the current explanation of the blue sky using scattering and the Raleigh equations reverses the mechanics, due mainly to this problem. Colorimetry is also affected , as well as explanations of the prism, of diffraction, and indeed of just about every problem in optics or QED. Three centuries of wave mechanics will have to be rewritten as spin mechanics.