Trig
Derivatives found without the old Calculus
including a Disproof of the Chain Rule.
Also the derivative of sin 5x
© Miles Mathis First
written October 2006, extended greatly June 2015 We
start with this equation, which is one of the defining trig
equations: y
= sinx
= ±√(1 - cos2x) Notice that
we are still dealing here with exponents. The cosine is squared
and that is the important fact here, not the fact that we are
dealing with trig functions. From a rate of change perspective,
the trig function is meaningless. A sine or cosine is just a
number, like any other. It is written as function of an angle,
but that does not affect the rate-of-change math at all. The
cosine of x
is a single variable, and we
could rewrite it as b
if we wanted to, to simplify
the variable for the rate of change math. Likewise, we could
rewrite sin x
as a,
if we desire. All we have to do is make sure we don't confuse
sine and cosine, since they vary in different ways, but we can
mark them anyway we want.
Let a
= sin x b
= cos x Therefore, we
could rewrite the equation as y
= a
= ±√(1 –
b2) Square both
sides y2
= 1 – b2 Since sine
and cosine are co-dependent, we can differentiate either side, or
both sides, starting with either side we like. Let z
= 1 – b2 z
= y2 Δz
= z'
= 2y
(from MM's table of exponents in
A Redefinition of the Derivative ) Δ(1 -
b2)
= 2y
Now switch
sides and differentiate again 2y
= Δ(1 – b2) 2Δy
= 2y'
= 2b
(once again, straight from the
table of exponents) y'
= b
= cos x You will say
that MM just followed normal procedure (a kind of chain rule), but
he did not, since whenever MM uses the equation nzn-1
= Δzn
he pulls it from table of exponents and constant differentials, not from
current sources, which MM has shown are all faulty. MM proves this
equation using a constant differential, not a diminishing
differential or a method using limits.
The table of exponents shows that with
the exponent 2, you only have to go to a third sub-change in the
rate of change chart in order to find a straight line, or a
constant rate of change. This means that you are not anywhere near
zero, and are not anywhere near an infinite series of any kind.
You are two steps below the given rate of change for this problem
(which is an acceleration or its pure math equivalent) and two
steps is two steps, not an infinite number of steps. In any rate
of change problem, we simply are not dealing with infinite series,
points, or limits. We are dealing with subchanges, and we are
seeking a line of constant differentials. Not a point, a line.
This is why MM's method is so important. It does not
matter in this problem that the curve was created by sine or
cosine. The way the curve was created does not concern us in
calculus. All we need is at least one dependence. If we have that
dependence then we can use the definition of exponent and integer
to create the table, and that table will straighten our curve out
in a definite and finite number of steps—the number of
steps being absolutely determined by the exponent itself. An infinite
series is only created by an infinite exponent. But an exponent
signifies a change, and a change requires time, so that an
infinite exponent would imply infinite time. We do not need to
solve equations concerning infinite time, not in physics and not
in mathematics. Therefore we have no need of infinite series in
rate of change problems. Here is the current and mainstream proof for the
derivative of (sinx)2.
To find this derivative, one normally uses the chain rule. To
apply the chain rule, you are instructed to first “take the
derivative” in the disallowed way. In other words, you just
drop the 2 down in front to find 2sinx
(similar to MM's proof but not the same). Next, you find the derivative
inside
the parentheses. Since the
derivative of sinx
is known to be cosx,
we multiply that by what we already have, obtaining the final
result 2sinxcosx.
To avoid the complaint that the
derivative of (sinx)2
is not
2sinx, the mainstream normally (or often)
does not
call that first step taking a derivative. Although it is
exactly the same manipulation, they just call it the first step
in the chain rule. Because they have given it a different title,
you are not expected to notice it is exactly the same
manipulation. Looking at MM's proof
of the derivative of sinx, you will notice
that to “find a derivative” here, we have to
“differentiate” twice, in both directions. That
should seem odd, since normally when we have a function to the
power of two, we only need to differentiate once
to find a derivative. We only
have to look at one rate of change, right? Well, you are not
taught it that way, but that is what is happening. But in this
functional equation we are looking at, we have both
sine and cosine, and both are
to the power of 2. We don't just have one function, we have two
interdependent or co-dependent functions, and both are squared.
And that is why we have to look at rates of change on both sides. Now,
admittedly, that is not the way these problems are normally
solved, but MM is claiming to solve them in a new way.
The old rules are sometimes wrong and
sometimes unnecessary, but sometimes they are necessary,
especially if you don't know what is going on with the rates of
change. In that case, you are best to stick to the old rules. But
if you know the difference between slopes, derivatives,
differentiation, and rates of change, you can simplify the math
considerably. To see this
in another way, notice that MM never claims that the derivative of
(sinx)2
is 2sinx.
only that that you can differentiate
y2
to find 2y, even while y =
sinx,
provided that you
do not stop there.
You must also differentiate in the other direction, because if
you don't you will not have related the two rates of change to
one another at the same time.
It is not that the derivative of (sinx)2
is 2sinx.
but that the rate of change of (sinx)2,
taken alone
or in isolation,
can be written as 2sinx.
However, since sinx
is, in fact, never found in
isolation, to find a derivative you have to keep going. You have
to find the rate of change of cosine simultaneously.
Thus one must differentiate in both directions.
Of
course the
first differentiation would not find any known derivative, since
it is only half a manipulation or half a relationship. Let me
continue. My critic might say, “Well, if that is so, why
can't you just insert (sinx)2
into the basic derivative
equation to find the real derivative? Why exactly is it allowed
in this interior differentiation, but not allowed in that direct
way?” Because if we are seeking the
derivative of
(sinx)2,
we are not just seeking the rate
of change of
sinx
by itself. We can only drop
the 2 down like that in cases where we are dealing with one rate
of change. However, as we have seen, sine and cosine are fatally
linked at all times. We saw it in the very first equation, sin2x
= (1 – cos2x).
And we see it after
we find the derivative of
sin2x,
which is 2cosxsinx.
That just tells us that whether you are given both or only one of
the two, you have to monitor both
rates of change. If you were
to just drop down the 2, you would not be monitoring both rates of
change, and you would get the wrong derivative. But MM is not
doing that in his proof, rather he is explicitly
monitoring the rate of change
in both directions (albeit in a somewhat compressed manner).
Which is why MM gets the correct derivative at the end (or the
absolute value, at any rate—the negative sign can be found
by monitoring the relative directions of change). Some
will not understand what is going on here, so here is
a simple diagram that might help. Cosine is
defined by that adjacent leg of the triangle, while sine is
defined by the opposite leg (both relative to the hypotenuse, of
course). If we draw the hypotenuse flat to the horizon, then we
can “see” the relative slopes of sine and cosine. If
we think of those upper legs like a roof, one slopes up while the
other slopes down, right? You may ask, how do we know which is
which? Aren't “up” and “down” relative
terms? Well, we have to put the triangle in some sort of
Cartesian graph, which specifies a direction. Once we do that,
with right indicating positive direction, say, then if one leg
slopes up, the other slopes down. Although this visualization is,
as usual, a simplification, it is useful when trying to
understand sine's relationship to cosine. This analysis
is borne out if we look at the
current mainstream proof that the derivative of sinx
is cosx.
Unlike the proof for (sinx)2,
the proof for sinx
cannot be found by any chain
rule, obviously. The proof is actually extremely long and
unwieldy, as you see if you take that link or study any other
similar site. Strictly, it is exactly as complex as the proof of
the calculus itself, since it relies on the same basic
“identity”—an identity that itself relies on
the infinitesimal h.
It is precisely this reliance on infinitesimals that MM is trying
to skirt around with his new method. At any rate, in this proof you can
see that sinx
can never be monitored by
itself. Every proof of sinx
must include cosx,
and the reverse. They are not just dependent functions, they are
interdependent
functions. Both have to be
monitored at the same time, because the slope of one is always
determined by both functions. Thus is why MM started with the
equation stating their interdependence, and why any
proof of the derivative of
either function must start with an equation that includes both. Now, to
find the slope of the opposite leg, say, rather than its length,
you can't just monitor sine alone. You can't build a triangle
with two sides, as the old saying goes. You also have to monitor
cosine. Why? Because if you are just given a term like sinx
and asked to differentiate,
you don't actually have enough information to solve and to mean
mean solve it as in find a specific number. It cannot be solved
because you don't have enough information to build a real
derivative equation or find a general slope equation. You don't,
because you are not given the length of the hypotenuse, for
instance, or even a variable for it. Without information about
the length of the hypotenuse, one leg of the triangle with its
angle is not enough to tell you any possible slope of sine or
cosine. Just consult the image above. Say you are given sinx.
That gives you possible values for x
and for the length of the
opposite leg, right? Now, if you also
have a possible value for
the length of the hypotenuse, you could write an equation for the
slope of sine, since you then have enough information to build
your triangle. Given all that information, there is only one way
you could draw in the adjacent leg to fit. In that case, for each
value of x
and sinx,
there is only one value of cosx.
But if you are not given any possible value for the hypotenuse,
you can't solve, since the adjacent leg could be any length. And
its length will then determine the slope of sine.
That is why
MM started with the equation sinx
= ±√(1 - cos2x)
in this paper (in both proofs), and why any proof of the slope or
derivative of either sine or cosine must start from an equation
that includes both.
MM will argue
that the chain rule (used in the current way) is the fudge, and
that his method is the correct one. You should be able to see that
applying a chain rule in this way to trig functions is actually
very slippery. We being to taught to basically make two
different manipulations on the same entity, but not being told
why. There is no chain here. There is only one link, that being
sinx.
Or, if you wish, we have two links, but both
of them are sinx.
We see that if we write the square out in long form: (sinx)(sinx) Why would you
take the derivative of one of those terms, but not the other? It
appears to me that we are actually taking the derivative of one
of them, then multiplying by a term that is not really the
derivative of both together. Why on earth would we wish to do
that?
To see what MM
means, notice again that when he solves these trig functions for
derivatives, he makes sure to manipulate both sine and cosine at
the same time. And yet when the mainstream applies the chain rule to (sinx)2,
they are not doing that. They are not looking at cosine
at all. They are trying to differentiate sinx twice, in
two different ways, which is why it certainly seems that they are
pushing the proof.
We see that
again by the fact the chain rule was meant to apply to a
functional relationship inside a functional relationship,
as in the equation Or the
equation
Please note that this paper is a simplification by me of a paper or papers written and copyrighted by Miles Mathis on his site. I have replaced "I" and "my" with "MM" to show that he is talking. All links within the papers, not yet simplified, are linked directly to the Miles Mathis site and will appear in another tab. (It will be clear which of these are Miles Mathis originals because they will be still contain "I" and "my".) The original papers on his site are the ultimate and correct source. All contributions to his papers and ordering of his books should be made on his site.
(This paper incorporates Miles Mathis' trig paper and trig2 paper.)

The derivatives of simple trig functions don't fit that form, as we can see even more clearly when they try to use the chain rule on a term like sin5x. They write it as sin(5x) and then try to use those imported parentheses to convince you they suddenly have a chain of functions. They tell you g(x) is 5x and sin(x) is h(x). But that is an obvious push, since to split the term that way implies sin5x can be written as two numbers. It can't. The sin5x is only one number or one variable. One number can't give you two functional relationships. There is no real “interior” and “exterior” here, since the sin signifier as written is neither a power signifier nor any other sort of multiplicative signifier. Sin with its variable is one term, and it cannot be split into two sub-functions. So the proof is just a fudge from the get-go.
Actually, the only reason you are being taught this faked mainstream proof is that it works. It is a push that gives you the correct result. And they have to push the proof because they don't understand what is really happening.
Now to explain what is really happening, and it has nothing to do with a chain rule (or not the given chain rule, at any rate). To discover it, we have to go back to MM's proof above, starting with the equation
(sinx)2 = 1 – (cosx)2
Since we are seeking the derivative of (sinx)2 instead of sinx, we need to start by differentiating the right side instead of the left. That gives us
Δ(sinx)2 = -2cosx
But, as we have seen, that can't be the full manipulation, since we have found a rate of change of cosx but not of sinx. We have monitored cosine for change but not sine. So what we need is the rate of change of (sinx)2 with respect to sinx. Or, to say it another way, we need to compare Δsin2 to sin. That is a sort of chain rule, but it is a very different chain rule than the one you are taught. To do that, we don't even have to differentiate: we simply divide the left side by sine, which gives us this:
Δ(sinx)2/sinx = -2cosx
That fraction, by itself, tells us how one term changes relative to the other. That is what a fraction is, in this case. You may think of it as one of those “with respect to” fractions if it helps you, but even that is not really necessary.
We can then move the sinx over to obtain this:
Δ(sinx)2 = -2cosxsinx
Since we know the rates of change are opposing, we can use that knowledge to drop the minus sign, giving us
Δ(sinx)2 = 2cosxsinx
Since in this case, my Δ is indicating the same thing as the current derivative (both are indicating a rate of change), MM's proof is complete since the finding matches the mainstream without using their fudged chain rule.
Also notice that each step has been explained. When have you ever been given a reasonable explanation of the chain rule as applied to this proof? There can be no reasonable explanation of the current chain rule as applied to this problem, because, as we have just seen, they totally ignore cosine in the proof. They manipulate sinx twice, in completely illogical ways. Cosine comes into the current proof only as a manipulation upon sine. In other words, they don't currently monitor any slope of cosine in order to solve. That cannot work, since to solve any trig derivative or slope, you have to monitor both sine and cosine. In my proof, MM monitors the change of sin2 against both cosine and sine, as shown.
Note the fact that the 2 actually enters the last equation above with the cosx, not the sinx. Which again proves the chain rule was a fudge in this case. We also see that the sinx in the equation is not the derivative of anything. It wasn't found by taking a derivative or by differentiating. It is just a straight relationship, as in any other fraction. You could say it that it is found by a form of calculus, since the relationship of sin2 to sine here is a “with respect to” relationship. But to say that it is not the derivative of anything is that we do not have to manipulate powers in any way. We just write the relationship as a fraction.
[A further clarification this proof is further down. There, MM tries to makes clear what some may already comprehend: MM is basically scaling his solution to sinx. So although it may look like he is illegally dividing only the left side by sinx, that is not what is happening. He is scaling a completed equality to an external function.]
So while it may seem MM has broken some great rules in his first proof of sinx, but really he has just used the given and longstanding manipulations in a more direct manner. In doing so, the old proof has been greatly simplified.
What is more important is that the mainstream is making up fake chain rules to suit themselves, and that they have been doing it for centuries. Although MM is not making a blanket argument against the chain rule here, but in this example it is clearly fudged. A bastardized form of the chain rule was used here to push this derivative of (sinx)2 to the known number. But, as we have seen, both manipulations in the chain rule were manufactured. In neither step were the proper relationships between functions discovered, and the 2 was completely mis-assigned.
It would be interesting to see who first fudged this proof of (sinx)2, and how long ago. It has probably been sitting out in the open since the time of Euler.
One might ask why MM can differentiate (sinx)2 to find 2sinx (as in my first proof), but the mainstream cannot. MM maintains that this chain rule was misused with this trig function because they created a fake chain by misreading the parentheses, and their failure to monitor both sine and cosine.
Derivative of sin5x
First published June 12, 2015
Previously, MM stated that the current proof of the derivative of sin 5x was pushed using the chain rule. Although MM gave a brief account of how it fails there, it will help to compare the pushed proof to a correct proof. This will also clarify the many problems with the current and historical proof.
MM showed previously that the chain rule was not really applicable to sin 5x because that cannot properly be fit to the chain equation:
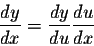
The relationship of sinx to sin 5x is not that kind of relationship. But even if someone could convince you that it is, the current proof would still be a push for a still more fundamental reason. That reason concerns the fact that applying the chain rule to this term fails to monitor the correct relationships. MM has shown that any time you are finding a derivative of sine, you also have to monitor cosine and therefore one has to take into account the relationship of sine and cosine (and their changes), and explicitly include it in your mathematical manipulations. Any proof that fails to do that is a fudge.
Therefore, to solve this problem or any other problem concerning trig derivatives, it helps to start with an equation that already includes both sine and cosine. You can't manipulate both if both are not already on the page. The mainstream sort of knows that, sometimes, since they have long solved for the derivative of sin by starting with an equation that also includes cosine. With that in mind, in that previous paper MM solved two basic trig derivatives by starting with the old defining equation
sin2x = 1 – cos2x
MM will do the same here. As you may have already noticed, he has now dropped the convention of writing that with parentheses, since the parentheses are just used by the mainstream to fudge the equation.
Now, if we are seeking the derivative of sin 5x , we can rewrite our first equation:
sin25x = 1 – cos25x
As before, we then differentiate the right side
Δsin25x = – 2cos5x
Again MM simply uses a delta to indicate change, rather than the current confusing notation, which is both varying and unwieldy. My notation—beyond being simpler and more direct— acts to remind us that we are actually representing the change of the functions on both sides; but on the left side we don't differentiate, simply representing the change with that delta. We do that to remind ourselves we are seeking the derivative of sine, not of cosine (and to remind ourselves that finding the derivative may take more than one step of differentiation).
So, we have monitored the change of cosine. Remember, the mainstream never does that in the current proof, which is one way we should have always known it was fudged. The mainstream manipulates sin 5x twice, but never manipulates cosine in any way.
Now, since both sine and cosine were squared in the first equation, we need to differentiate sin2 as well. But as we do that, let's look ahead to see what else we need to do. Since we started by substituting 5x for x in the first equation, we need to look at how that will affect our solution. We are allowed to do that to suit ourselves, but we can't do it and then ignore the consequences. For instance, if we originally had x = 45 , say, then after we make the change, 5x also has to equal x = 45 . Which makes x now equal to 9. If we do that, we see that sinx and sin5x are not really scaling to one another. We need them scaled to one another, since we need to be able to relate them both to the same number line, which is of course based on the number 1. But by solving for 5x instead of x, we have thrown that scaling off. We won't be able to compare rates of change of the two trig functions directly unless we scale them to one another.
Obviously, that is very easy to do, since the scaler is just the number 5. But how do we legally work in that scaler? Well, there are various ways to do it, but one way is to work it into the rate of change math. Like so:
Δsin25x = – 2cos5x
ΔΔsin25x /Δsin5x {with respect to Δsinx } = – 2cos 5x
This time we don't put a delta in front of the right side, because we have already found the change over there. This second differentiation is not really the reverse of the first differentiation, since the first differentiation was the change of cos2 relative to sin2. We don't need to reverse that, since we don't need to know the change of sin2 relative to cos2. Once we know the relationship in one direction, we know all we need to know in that regard. Rather, the “reverse” differentiation is the change of sin2 relative to sin (while importing the scaler). So we have to track deltas only on the left side.
MM is writing out the expanded proof here, instead of the compressed proof written for sin<x previously. This to show that although you basically just differentiate both sides, you differentiate sin2 for a different reason than you differentiate cos2. You can see that this is very important in this case, because the denominator doesn't reduce to 1 here, as it did (or would have) with the proof of sinx. We use the denominator to import the scaler.
Concerning the actual operation: when you differentiate a term, you take away one of the deltas, since each delta is telling you that you can differentiate. After you actually differentiate, you don't need it anymore. So we are down to this:
Δ2sin5x/5 = – 2cos5x
Δsin5x = – 5cos5x
This also shows us where the 5 comes from in the final equation. The mainstream derives that 5 by differentiating 5x as the second part of the chain rule, but you can now see how that was a fudge. You can't differentiate 5x separately since it is not an interior part of the chain in that way. You can't separate it from its sin or cos signifier. And you don't need to. Strictly speaking, the number 5 doesn't even come from the rate of change math in the same way as the rest (which is yet another reason the chain rule is inapplicable here). It is imported into the equation after the fact, as a scaler. MM has imported it as a rate of change to match it to the other notation, but you could just as easily import it without differentiating anything, as a raw scaler or constant. Since sin and cosine change in opposite ways as we did earlier, we can drop the negative sign, obtaining this final equation:
Δsin5x = 5cos5x
Now to answer a couple of questions. Some of you may understand that my proof is still somewhat compressed, since we have to be careful how we treat those two deltas in the numerator on the left side. Some will say, “Why not just differentiate the numerator first in the second step, since then you would get 2Δsin5x/Δsin5x? That would then reduce to 2.” The reason you can't do that is because the second delta in the numerator is telling us that we are relating changes between the numerator and denominator. The deltas on top and bottom go together and have to be solved together. So you can't differentiate the numerator and then just stop. You have to differentiate them together, because we are monitoring how they change relative to one another. If you just differentiate one, you won't have discovered that, and your manipulation will be in vain. To indicate that, we probably need to tweak our notation just a bit. This might do it:
Instead of ΔΔsin25x /Δsin5x = – cos5x
Write it as ΔΔ'sin25x /Δ'sin5x = – cos5x
Various other simple notations might work as well. The second question would go something like this: “In the second step, you divide only the left side by Δsin5x . How is that legal? Shouldn't you have to divide both sides by the same amount?” No, because, as was shown, that entire manipulation was done outside the given equality, as a scaler to a function outside the equation. We are scaling here to sinx , which never appears in the equation. And we are doing that so that we can compare the derivatives of sinx and sin5x directly after we solve.
You might ask, “Well, is not that what the mainstream is doing with the chain rule?” In effect, yes. In theory, no. The mainstream instructs you to take the derivative of 5x because it is inside the parentheses. But that is not why you do it. The parentheses have nothing to do with it, and there no interior in that way. You do it to scale the final equation to sinx, and they never tell you that.
Then one might ask, “Well, even if it is a scaler, shouldn't you import it on both sides? Aren't you still breaking a rule?” No, because there is no way to import a scaler on both sides of an equation. You can only import it on one side or the other, and you import it where it logically goes. In this case, it logically goes on the sine side of the equation, since we are scaling to sinx.
Like the chain rule, MM has two main manipulations here. So although you might call his solution a sort of chain rule, it is very different than the current chain rule regarding trig functions. Notice that in the current solution, no mathematical manipulations are done upon cosine. Cosine only enters as the derivative of sine. But in his proof, MM makes it clear that cosine has to be in the solution from the start. You will tell me that cosine is in the current proof implicitly, since to find the derivative of sin x the mainstream also manipulates cosine. But that manipulation is also a fudge, as MM has shown with powers in The Proof for the Current Derivative for Powers is False.
The current method for finding the derivative of sinx is quite complex, and it relies on misusing infinitesimals or infinite series, in precisely the same way as in the fundamental proof of the infinite calculus. All you have to notice is how they are pushing the term h there, to understand how the entire proof is pushed. They push the proof of sinx just as the push the proof of the calculus itself.
We can see that by the way cosine enters my equation. In the current proof of the derivative of sin5x , cosine enters as the derivative of sine. In my proof, cosine enters as the differential of cos2 . That is a big difference. We saw a similar thing previously, where MM pulled apart the current proof of the derivative of sin2x . In that case, we saw the number 2 entering the final equation from the wrong place, proving the current proof was fudged. Here we saw the number 5 entering from the wrong place, proving the same thing.
As MM compiles more and more of these specific pushes, it confirms more and more my basic contention that the foundations of the calculus have never been understood. If mainstream mathematicians had ever understood how these changes were working, or where they were coming from (see the table in my long paper ), these pushes would have been impossible. But these pushes in the trig functions make me think that trigonometry has also been opaque in some ways at the foundational level up to the present time. If mathematicians really understood how sine and cosine were linked in an operational— and one might say physical —way, they would never have made the mistake of manipulating one without the other.
Modern mathematicians have proven themselves adept at manufacturing increasingly abstract and complex number systems and manipulating those systems in any way that suits them; but when numbers get near any physical situation, those same mathematicians have proven themselves very poor practitioners. Or, to say it another way, whenever math becomes applied math, the applications suddenly become slippery in the extreme. This can only be due to the fact that the mathematicians don't understand what their numbers actually apply to.
Trigonometry is the perfect proof of this, since trig is always an applied math. At its most abstract, it is applied to drawn figures, but at it most useful, it is applied to real objects and situations. But in either case, it is applied. It is never pure math. Perhaps this knowledge of sine and cosine was being taken for granted—the true extent of their interdependence—was not actually known. Perhaps most mathematicians and physicists had never considered the impossibility of monitoring sine without cosine. Not specializing in the history of science or math, MM cannot say that no one has known this, but it does seem curious that those who knew it would find trig derivatives the way they are currently found. Since they are currently found the same way they have always been found—roughly—it must mean that mainstream mathematicians and physicists haven't fully comprehended all the physical implications of either trigonometry or calculus, all the way back to Newton and Leibniz. That is not too surprising, considering the fact that applied math is still in its infancy, having been applied to complex engineering feats for only a few centuries. Still, the idea goes against all we are taught, since we are taught that math and physics are well nigh perfect and finished. Clearly there is a lot more to learn.